Solving the mathematical equation is usually the solution to most of the problems faced by the math students. However in the real life other practical methods exist to find the circumference of a circle. Imagine finding yourself in the need of calculating the circumference of a circular ring.
Obviously it would be difficult to find the exact radius or diameter of such and object. In this case the simple solution is to make a mark on the ring and place it so that the mark touches the floor. Then we place another mark on the floor in the exact place where we have the mark on the ring. Next it is enough to roll the ring to the point when in makes the full revolution and the mark ends directly on the floor again. We make another mark in that place on the floor.
Now we can simply calculate the distance between the two marks on the floor using simple tape measure. This distance will be equal to the circumference of the given ring. Rolling the ring we have to make sure the ring does not slide as this would falsify the result of the measurement. We'll teach you the key circumference formulas you need to figure out the circumference of a circle when you know either the diameter or radius. The diameter of a circle is twice to that of the radius. If the diameter or radius of a circle is given, then we can easily find the circumference.
We can also find the diameter and radius of a circle if the circumference is given. We round off to 3.14 in order to simplify our calculations. Circumference, diameter and radii are calculated in linear units, such as inches and centimeters. A circle has many different radii and many different diameters, and each one passes through the center.
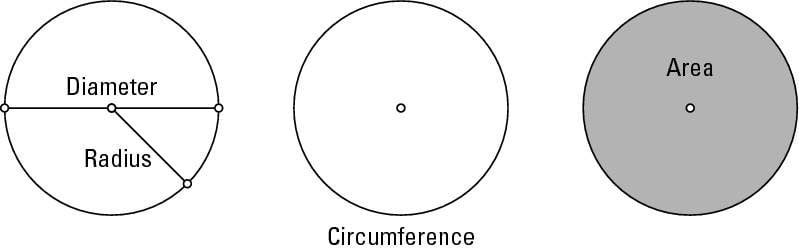
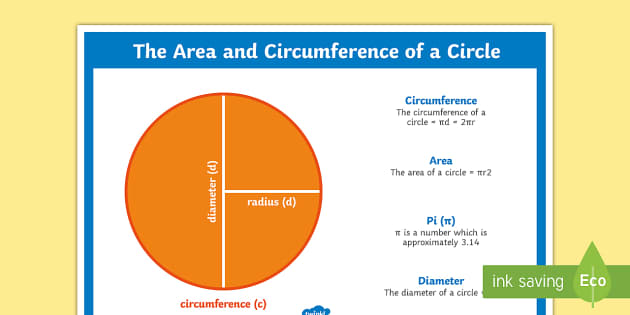
A circle is a geometric form of which every point on the outside of the circle is the same distance away from the center. The distance around the edge of the circle is called the circumference. The distance from one side of the circle to the other, going through the center of the circle, is the diameter. The constant pi, designated by the Greek letter π, is the ratio of the circumference to the diameter of a circle. For any circle, if you divide the circumference by the diameter you get pi, an irregular number usually rounded to 3.14. The circumference of a circle of radius $r$ is $2\pi r$.
This well known formula is taken up here from the point of view of similarity. It is important to note in this task that the definition of $\pi$ already involves the circumference of a circle, a particular circle. In order to show that the ratio of circumference to diameter does not depend on the size of the circle, a similarity argument is required.
Two different approaches are provided, one using the fact that all circles are similar and a second using similar triangles. This former approach is simpler but the latter has the advantage of leading into an argument for calculating the area of a circle. To understand how to calculate circumference we must first begin with the definition of circumference. Circumference of a circle is linear distance around outer border of a circle. To find out the circumference, we need to know its diameter which is the length of its widest part. The diameter should be measured in feet for square footage calculations and if needed, converted to inches , yards , centimetres , millimetres and metres .
Life is full of circles (and semi-circles). Therefore, the equation of the circumference of a circle can be useful in solving any problem involving circles. Since circumference is a measurement of length, there are generally two main kinds of problems that will involve calculating the circumference of something circular. First, knowing the circumference of a circle can be used to create something circular.
Second, if the circle is moving at a known speed, then the circumference can be used to determine how far the circle might travel in a given time. It looks like you calculated the area of a circle using a radius of 2; in this figure, the radius of each circle is 1. To find the area of the figure, imagine the two semi-circles are put together to create one circle. Then calculate the area of the circle and add it to the area of the square.
The formula for calculating the circumference of a circle from the diameter of a circle is very easy. Simply multiply the diameter by the number 3.14, which is also called the number pi (π). The answer will be in the units of length by which the diameter is measured. The radius, the diameter, and the circumference are the three defining aspects of every circle. Given the radius or diameter and pi you can calculate the circumference.
The diameter is the distance from one side of the circle to the other at its widest points. The diameter will always pass through the center of the circle. You can also think of the radius as the distance between the center of the circle and its edge. To calculate the diameter of a circle, multiply the radius by 2.
If you don't have the radius, divide the circumference of the circle by π to get the diameter. If you don't have the radius or the circumference, divide the area of the circle by π and then find that number's square root to get the radius. Then, you can just multiply the radius by 2 to find the circle's diameter.
The first solution requires a general understanding of similarity of shapes while the second requires knowledge of similarity specific to triangles. The distance from the centre to the outer line of the circle is called a radius. It is the most important quantity of the circle based on which formulas for the area and circumference of the circle are derived. Twice the radius of a circle is called the diameter of the circle.
The diameter cuts the circle into two equal parts, which is called a semi-circle. Lauren is planning her trip to London, and she wants to take a ride on the famous ferris wheel called the London Eye. While researching facts about the giant ferris wheel, she learns that the radius of the circle measures approximately 68 meters. What is the approximate circumference of the ferris wheel? Worksheetto calculate circumference of circle when given diameter or radius. It looks like you calculated the area of the square, but not the circle.
Imagine the two semi-circles are put together to create one circle. Find the total perimeter by adding the circumference of the semicircle and the lengths of the two legs. Since our measurement of the semi-circle's circumference is approximate, the perimeter will be an approximation also. Now that you know how to calculate the circumference and area of a circle, you can use this knowledge to find the perimeter and area of composite figures.
The trick to figuring out these types of problems is to identify shapes within the composite figure, calculate their individual dimensions, and then add them together. Not just this but there are some significant distances on a circle that needs to be calculated before finding the circumference of the circle. Diameter is the distance from one side of the circle to the other, crossing through the center/ middle of the circle.
For example, the length of a 180 degree arc must be half the circumference of the circle, the product of pi and the radius. The length of any arc is equal to whatever fraction of a full rotation the arc spans multiplied by the circumference of the circle. A 45 degree arc, for example, spans one-eighth of a full rotation, and is therefore equal to one-eighth the circumference of that circle.
The length of an arc of n degrees equals (n/360) times the circumference. As stated before, the perimeter or circumference of a circle is the distance around a circle or any circular shape. The circumference of a circle is the same as the length of a straight line folded or bent to make the circle. The circumference of a circle is measured in meters, kilometers, yards, inches, etc. Learn the circumference equation and definition. See examples of how to calculate circumference when given the diameter or radius.
Once again in this example, we're given the radius of the circle. Although it's not a clean number like our previous example, but we can still simply plug the number directly into the formula like what we did above. Be aware of the units that this circle's radius is given in and remember to give your final answer in the same unit. In this question, we find that the circumference is equalled to 53.41m. When we use the formula to calculate the circumference of the circle, then the radius of the circle is taken into account.
Hence, we need to know the value of the radius or the diameter to evaluate the perimeter of the circle. You can think of it as the line that defines the shape. For shapes made of straight edges this line is called theperimeter but for circles this defining line is called the circumference. The circumference of a circle is the distance around the circle and it's equal to ???
The importance of pi has been recognized for at least 4,000 years. By the start of the 20th century, about 500 digits of pi were known. With computation advances, thanks to computers, we now know more than the first six billion digits of pi. The diameter of any circle is two times the length of that circle's radius. Blame Welsh mathematician William Jones for the trouble pi causes you.
In 1706 Jones used the Greek word for circumference, περιϕέρεια, and shortened it to its first letter, π. As for π in circles, we have no one to blame but the marvel that is mathematics. Pi, or π, is the irrational number that links every circle's circumference to diameter and radius.
Using geometrical formulas and equations gets easier with practice. Ask for help from someone who has worked with circles or any other geometrical figures. You're likely to find that questions of geometry will seem less challenging with a little experience. The diameter of a circle can be calculated according to the given parameters. If the parameters like radius, circumference, or area are given then we can directly use the following formulas. The diameter is defined as twice the length of the radius of a circle.
This first argument is an example of MP7, Look For and Make Use of Structure. The key to this argument is identifying that all circles are similar and then applying the meaning of similarity to the circumference. The second argument exemplifies MP8, Look For and Express Regularity in Repeated Reasoning. Here the key is to compare the circle to a more familiar shape, the triangle.
Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations. Worksheetto calculate circumference and area of circle when given diameter or radius. Calculating the circumference of a circle involves a constant called pi, with the symbol π. Pi (π) is the ratio of the circumference of a circle to its diameter. Pi is always the same number for any circle.
One interesting property about circles is that the ratio of a circle's circumference and its diameter is the same for all circles. No matter the size of the circle, the ratio of the circumference and diameter will be the same. The word 'perimeter' is also sometimes used, although this usually refers to the distance around polygons, figures made up of straight line segments.
Circle itself by its definition is a simple & closed shape. It is a set of all points in a plane that are of the same distant from a given point, called as the center. It can also be known as a curve, outlined by a point where the distance from a given point remains same as the point changes. While a circle, symbolically, signifies various things to different people that include the concepts like infinity, persistence, and entirety. If you know the circumference, radius, or diameter of a circle, you can also find its area. Area represents the space enclosed within a circle.
It's given in units of distance squared, such as cm2 or m2. If you have the measurement of the circumference of a circle, divide it by π and you will get the diameter. The value of π is approximately equal to 3.14 but you must use the calculator to obtain the most accurate results. As an example, if the circumference of the circle is 25 cm, then the diameter is 25 cm/π, or 7.96 cm. There is just one diameter drawn on circle C.
Even though every circle has an innumerable number of possible diameters. Imagine you have to cut a round pizza into two equal pieces. Nevertheless, how you turn the pizza, as long as you make one straight cut right through the center point of the pizza, you will divide it along a diameter. Fizzywoz - If you look at examples #1-#4 at the end of the article you can see how numbers are plugged into the equation.
You will usually just plug in a known radius into the equation to figure out the circumference or you may plug in a known circumference to determine the radius. Let me know if you have any other questions. Check out 4 common types of geometry homework problems and solutions involving the circumference of circles.










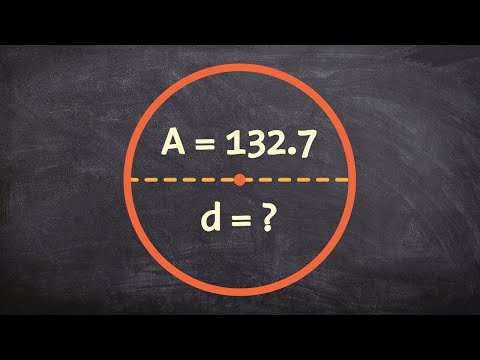















No comments:
Post a Comment
Note: Only a member of this blog may post a comment.